Logic: On the lighter side

Alice C. Linsley

Pick your stick figure: Clean-Shaven or Goatee Guy. Clean-Shaven states that Modal Logic is "special" and gives attributes which we are to assume make Modal Logic "special."

Goatee Guy does most of the talking. He uses words like complex, claim, conclusion, problem.

Are these two figures in the same world metaphysically speaking?



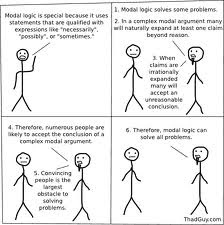
1.Clean-Shaven says, "Modal logic is special because it uses statements that are qualified by expressions like 'necessarily', 'possibly', or 'sometimes.' Modal logic solves some problems."

2. Goatee Guy says, "In a complex modal argument many will naturally expand at least one claim beyond reason."

3. Goatee Guy says, "When claims are irrationally expanded many will accept an unreasonable conclusion."

4. Goatee Guy says, "Therefore, numerous people are likely to accept the conclusion of a complex modal argument."

5. Goatee Guy says, "Convincing people is the largest obstacle to solving problems."

6. Goatee Guy says, "Therefore, modal logic can solve all problems."


So which is it? Modal logic solves some problems or modal logic solves all problems.

Can both be valid claims? Would this be allowed by Aristotle's First Principles? What about the Laws of Identity and Non-Contradiction?

In what possible world would such contradiction possibly exist? Under what conditions? (Think about our enactment of the courtroom scene with the different witnesses. Think of the contrast between the naked spear-throwing Amazon native who laughs at the idea of a man walking on the moon and the rich American who pays for a trip to the moon.)


Saul Kripe

Saul Kripke began his work on the semantics of modal logic while he was in high school. His groundbreaking paper, “A Completeness Theorem for Modal Logic,” was published in the Journal of Symbolic Logic in 1959 during Kripke’s freshman year at Harvard.

Kripke’s most important publication, Naming and Necessity (1980), was based on three lectures he delivered at Princeton in 1970. The book provides an account of necessity and possibility as metaphysical concepts. It distinguishes necessity and possibility from a posteriori knowledge and a priori knowledge and from the semantic notions of truth by virtue of meaning and truth by virtue of fact. In the course of making these distinctions, Kripke revived the ancient doctrine of essentialism, according to which objects necessarily possess certain properties without which the objects would not exist. Here Kripe goes back to ontology. He maintains the existence of contingent a priori truths, to which Michael Dummett reacts, maintaining that there is something wrong with Kripe's logic.

In modal logic claims are said to be deeply contingent or superficially contingent.


Necessary versus contingent

Analytic propositions are the focus of Analytical Philosophy and Modal Logic. An analytic proposition is a statement or judgment that is necessarily true on logical grounds and serves only to elucidate meanings already implicit in the subject. The truth of such a proposition is guaranteed by the principle of contradiction. An analytic proposition is distinguished from a synthetic proposition which derives meaning from nonlogical and/or sense data which, as Bacon, Berkeley and others have demonstrated, are contingent upon the one sensing and their circumstances.

To illustrate this distinction, think of Descartes' proposition that all bodies are extended. This is an analytic proposition because the idea of extension is implicit in the notion of body; whereas the proposition that all bodies are heavy is synthetic, since in addition to the notion of body, the notion of weight supposes the notion of bodies in relation to one another. Without a point of reference, "heavy" means nothing and with a point of reference "heavy" is synthetic.

In the 19th century Bernard Bolzano added a third category, the analytically false. He held that a sentence is analytically true if either (1) its propositional form is true for all values of its variables or (2) it can be reduced to such a sentence.

Gottfried Wilhelm Leibniz made a parallel distinction between “truths of reason” and “truths of fact,” and David Hume distinguished between “relations of ideas” and “matters of fact.”

In the mid-20th century, the distinction between analytic and synthetic statements stirred extensive debate in view of objections raised by the American logician Quine. Quine also goes back to ontology in his statement: "To be is to be the value of a bound variable."

Quine once heard a talk at Harvard by a famous philosopher. Afterwards Quine was asked what he thought of the talk, and he replied, “He paints with a broad brush.” Then he added, “and he thinks with one too!” Quine's mind was such that he must have regarded the majority of humans to be mired in ambiguity.


Immanuel Kant

Having been aroused from his philosophical slumber by David Hume, Immanuel Kant made a distinction between statements whose predicate is included in the subject (analytic statements) and statements whose predicate is not included in the subject (synthetic statements). The distinction was introduced by Kant in The Critique of Pure Reason.

For Kant, "predicate" refers to what can be said or predicated about something. Anything that can be publicly declared and asserted about something is a predicate. Kant called an attribute, property, quality, or characteristic that can be predicated about something a "category." Kant's category does not mean classification, but merely some object generally known. Kant wrote, "…I remark concerning the categories…that their logical employment consists in their use as predicates of objects." He called them "ontological predicates."


Related reading: Theories of Change and Constancy; Liebniz' Modal Metaphysics; Modal Logic and Problem Solving; Why Logic should Be Taught in Schools; Introduction to Logic: Fallacies; Pioneers in the Field of Logic